Cubic Formula Proof Step 4: Other Solutions of y using the Cube Root of Unity
In this video I go over the fourth step of the Cubic Formula proof which involves obtaining the complex solutions of y by considering the cube root of unity. Since the cube root of the z values from Vieta's substitution has 3 factors, we need to multiply the principle solution by the factors to obtain each separate solution. We solved the first solution already in the previous video, and that solution is termed the principle solution since it corresponds to the factor 1. The next two factors are complex numbers, but when we multiply them by z, we can apply the inverse relationship to simplify the equations. The result is just the principle solution of y with each term multiplied by one complex factor; the order is flipped for the other solution.
In the next video, I will plug these y-values back into the PQ substitution to obtain our x solutions.
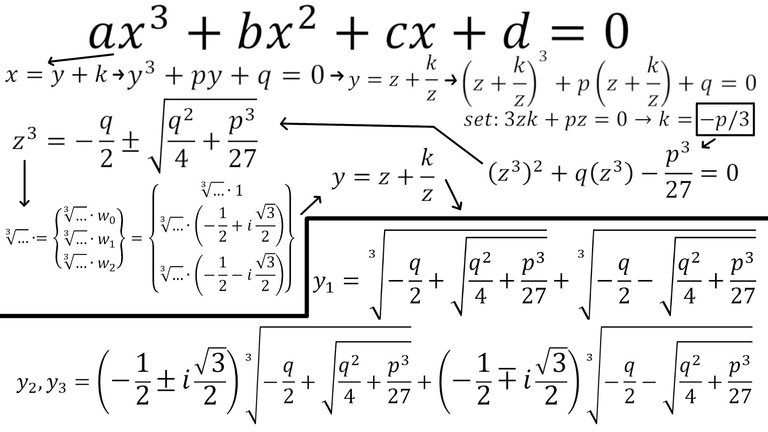
Timestamps
- Step 4: Find the other solutions of y using the cube root of unity: 0:00
- First solution is just our previous or principle solution of y: 2:00
- Next two solutions involve multiplying by the complex roots: 2:36
- Solving the second solution of y: 3:26
- Solving the third solution of y: 6:28
Notes and playlists
- Playlist: https://www.youtube.com/playlist?list=PLai3U8-WIK0EF05ExjzLbB64NgUjoV1hl
- Notes: https://peakd.com/hive-128780/@mes/dzekfnxh .
Become a MES Super Fan! https://www.youtube.com/channel/UCUUBq1GPBvvGNz7dpgO14Ow/join
DONATE! ʕ •ᴥ•ʔ https://mes.fm/donate
SUBSCRIBE via EMAIL: https://mes.fm/subscribe
MES Links: https://mes.fm/links
MES Truth: https://mes.fm/truth
Official Website: https://MES.fm
Hive: https://peakd.com/@mes
Email me: [email protected]
Free Calculators: https://mes.fm/calculators
BMI Calculator: https://bmicalculator.mes.fm
Grade Calculator: https://gradecalculator.mes.fm
Mortgage Calculator: https://mortgagecalculator.mes.fm
Percentage Calculator: https://percentagecalculator.mes.fm
Free Online Tools: https://mes.fm/tools
iPhone and Android Apps: https://mes.fm/mobile-apps
▶️ 3Speak
!summarize
Part 1/5:
Finding Multiple Solutions with Cube Roots of Unity
In our journey into the world of complex numbers and cube roots, we have reached a pivotal stage where we explore the application of cube roots of unity to discover multiple solutions for the equation involving an unknown variable ( y ).
Understanding Cube Roots of Unity
To start, cube roots of unity are solutions to the equation ( x^3 = 1 ). These roots are crucial when working with cubic equations and allow us to break down complex problems into simpler components. In essence, they give us three distinct solutions: 1 (the principal root), and two other complex roots, typically denoted as ( W_1 ) and ( W_2 ).
The Primary Solution
Part 2/5:
We begin by considering one of the cube roots ( z ), expressed as ( \sqrt[3]{Z} ). By applying the cube roots of unity, we can generate three solutions for the variable ( z ):
The principal cube root, denoted as ( z_1 ), which is equal to ( \sqrt[3]{Z} ).
The second solution, ( z_2 ), is represented as ( z_1 \times W_1 ), where ( W_1 ) is one of the cube roots of unity.
Finally, the third solution, ( z_3 ), is derived as ( z_1 \times W_2 ), introducing the last cube root of unity into our calculations.
Converting ( z ) Solutions to ( y ) Solutions
Part 3/5:
Having identified our three solutions for ( z ), we can now substitute these values into the related equation to solve for ( y ). The original equation, ( y = \frac{z - p}{3z} ), serves as our framework for transformation.
Calculating ( y_1 )
Starting with ( z_1 ):
[
y_1 = \frac{z_1 - p}{3z_1}
]
This utilizes ( z_1 ) directly in our computation.
Progressing to ( y_2 )
Next, we substitute for ( z_2 ):
[
y_2 = \frac{z_2 - p}{3z_2}
]
By reorganizing, this can be expressed through ( z_1 ) as follows:
[
y_2 = \frac{(z_1 \times W_1) - p}{3(z_1 \times W_1)}
]
Further simplification leads us to recognize relationships between ( W_1 ) and ( W_2 ), allowing us to express ( y_2 ) with respect to our existing terms.
Finally, ( y_3 )
Part 4/5:
Similarly, we compute ( y_3 ):
[
y_3 = \frac{z_3 - p}{3z_3}
]
Replacing ( z_3 ) with ( z_1 \times W_2 ):
[
y_3 = \frac{(z_1 \times W_2) - p}{3(z_1 \times W_2)}
]
This equation mirrors the complexity of ( y_2 ), demonstrating how the intricate relationships between cube roots translate back into the quotient required for ( y ).
Conclusion
Through the methodical breakdown of cube roots and their application to complex equations, we have successfully modeled the relationships between ( y ) and its corresponding ( z ) values guided by cube roots of unity. Each derived solution illustrates the elegance of algebraic manipulation and the beauty inherent in solving cubic equations.
Part 5/5:
As we continue to explore mathematical concepts, the techniques discussed here empower us to tackle increasingly sophisticated problems, using fundamental principles of unity, complex numbers, and cubic functions.